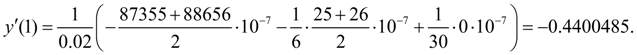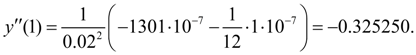Contents >> Applied Mathematics >> Numerical Methods >> Numerical Differentiaon >> Example

Numerical differentiaon - Example

Example [1]

Find у ’ (1)  and у ’’ (1) for function у ( х ), given tabular:

Values of function у ( х )

x

y

Δy

Δ 2 y

Δ 3 y

Δ 4 y

0.96

0.7825361

– 86029

0.98

0.7739332

– 1326

– 87355

25

1.00

0.7651977

– 1301

1

– 88656

26

1.02

0.7563321

– 1275

– 89931

1.04

0.7473390

S o l u t i o n .  According to the table we’ll receive: h = 0.02, х = 1, х 0 = 1, hence, q = 0. Having made final differences of function у ( х ) and using underlined values, from formula (9) we’ll receive:

Similarly, using blacked out values from formula (10) we’ll receive:

For comparison with exact values we’ll notice, that tabulated function is Bessel’s function at which the first derivative at х = 1 is equal to – 0.4400506, and the second, accordingly, – 0.325147. From here it is visible, that numerical finding of the second derivative gives greater error, than for the first derivative.


< Previous Contents Next >