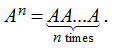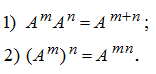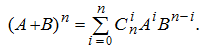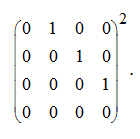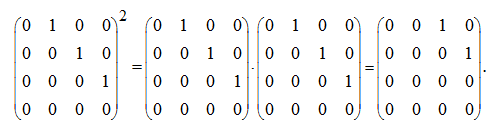Powers of matrices

Let A – a square matrix and n – a natural number. Then the n -th power of the matrix A is:

Besides it is considered, that , where Е – an identity matrix.
If A is a regular (non-singular) matrix, it is possible to enter a negative power of a matrix:

For powers of matrices with the whole indices the following rules take place:

If A and B – square matrices of the same orders, and АВ = ВА , then Newton’s binomial formula takes place:

E x a m p l e  [1] .  Find

S o l u t i o n .