Contents
>> Engineering Mathematics
>> Control Systems
>> Dynamic Synthesis of Control System of Hydraulic Drive
>> Problem statement
|
Problem statement
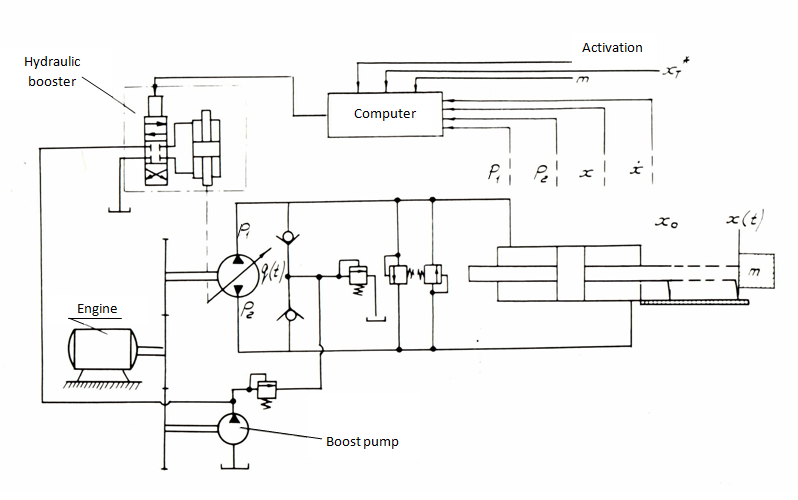
Level of realizable capacities, high power consumption, indispensability to carry out regulation over a wide range, operating conditions, a well-established batch production of the appropriating equipment allows to consider, that as the most suitable type of a power drive for positioning executive mechanisms, for example, building manipulators the volumetric hydraulic drive with volumetric or throttle regulation is. The primary problem of control algorithms is maintenance of purposeful movement of the executive mechanism. Now there are very much powerful tools making a methodological basis of the control theory [1, 3 – 7]. In monographs [3, 6, 7] the mathematical theory of optimum control which is based on the maximum principle of Pontryagin is presented. In the most full measure methods of the optimum control using this principle, are developed for linear systems [8] for which the principle of a maximum is at the same time a necessary and sufficient condition of an optimality. For nonlinear systems (to which the hydraulic drive concerns also) application of this apparatus appears much less effective as the class of problems for which synthesis of optimum control we’ll carry out analytically is very narrow. In the majority of cases it is necessary to establish, first, sufficient conditions of optimality for each specific problem, and secondly, construction of optimum decisions is possible (by virtue of nonlinearity) only numerical methods that brings to nothing practical advantages of the given theory. So, in case of hydraulic system with volumetric regulation use of a principle of a maximum becomes impossible because of the insuperable analytical difficulties connected both with restrictions-nonlinearities of phase coordinates of hydraulic system, and with unsolvability of system of the conjugate differential equations because of availability in them of initial phase coordinates (as consequence of nonlinearity of mathematical model of a hydraulic drive). Application of numerical methods does not rescue position as the enormous volume of the calculations necessary for the solution to the problem, will lead in an actual control system to irreplaceable expenses of time. In the monograph [5] questions of stability software controlled motions, including their construction, as well as methods of synthesis of controls, including optimum are considered. The approach to a finding of necessary and sufficient conditions of optimality in various variation problems is under construction on the basis of Lyapunov's second method. However the statement has especially theorized character, little fit for practical use in considered problems of control movement of executive mechanisms. Essentially other approach at construction of control algorithms for manipulators and robots is based on a method of movement trajectory planning for the executive mechanism [1, 4]. The generalized coordinates of executive mechanisms in control systems of manipulators and robots usually get out so that each separate servo drive operated variation of one generalized coordinate. Therefore desirable laws of variation of generalized coordinates, defined at a stage of planning, at the same time are operating signals of these drives. In a mode of automatic planning on a computer these problems should be solved by means of special algorithms which possible versions are considered in the monograph [1]. In the given section some control algorithms with reference to systems of a power hydraulic drive are considered. The control system which on the one hand in a condition to process great volume of the entrance information on parameters of the machine is necessary for their realization, and with another – on the basis of this processing by means of special algorithm to choose optimum (by this or that criterion) control. The decision of such problem should be carried out for possible minimal time. Thus, statement of the problem is maybe stated as follows. Object of researches are systems of a power hydraulic drive of volumetric and throttle regulation with reference to building manipulators both the robotized machines and arrangements. By manufacture building and installation works according to existing specifications high accuracy of positioning of the great mass cargo is necessary. Besides on technology of works on construction at moving cargoes realization enough complex trajectories with overlapping working operations of building machines and mechanisms is required. The problem of formation of movement control algorithm on some degree of mobility of the mechanism generally is maybe stated as follows: – to provide moving a cargo (variable mass) from initial position x 0 in final position x Т * for minimally possible time with the maximal accuracy at compliance of some restrictions (on speed, acceleration, pressure). It is represented, that the most appropriate decision of a task in view will be a combination of two stages of control: 1) control of the opened system according to the given law of movement [1] with tracking current value of the operated coordinate – so-called "rough" control . Thus the given accuracy of positioning can not be reached, but the greatest possible speed should be provided; 2) control of the closed system with connection of appropriating correcting links by position, speed and acceleration at achievement in current coordinate of the value close to target – so-called "precise" control . Thus the choice of feedback should provide not only the given accuracy of positioning, but also aperiodicity of process in a zone of regulation and a necessary stability factor. Thus, at positioning executive mechanisms there is a problem of construction of movement control algorithm of servo drive with feedback on phase coordinates (for a hydraulic drive of progressive motion – on moving x ( t ) and speed v ( t ) of the hydraulic cylinder piston, for a hydraulic drive of rotary motion – on angle of turn φ ( t ) and angular speed ω ( t ) of shaft (of hydro motor, reducer, rotary platform), as well as – to pressure p 1 ( t ) and p 2 ( t ) in pressure and drain cavities of a hydraulic engine). On Fig. 1 the basic circuit diagram of a power hydraulic drive of volumetric regulation with the closed circulation of a stream is presented.
Fig. 1. Phase coordinates
measured during movement move on an input of computer by means of which the algorithm of optimum control by movement is shaped. Control in the
given diagram is the working volume
q
(
t
) of the variable displacement pump, changed by means of the hydraulic booster on which
input the operating signal from a computer moves. A feed of the hydraulic booster and the compensation of leakages in hydraulic system are carried
out by the boost pump. For the considered diagram the problem of formation of optimum control algorithm for a power hydraulic drive is stated as
follows.
To provide control q ( t ) for a drive of movement of a cargo mass m from a point x 0 in a point x Т * for minimal time Т at the restrictions: 
where x Т , x Т * – accordingly the actual and given final position of a cargo; 3) | q ( t ) | ≤ q p , (3) where q p – the maximal working volume of the pump.
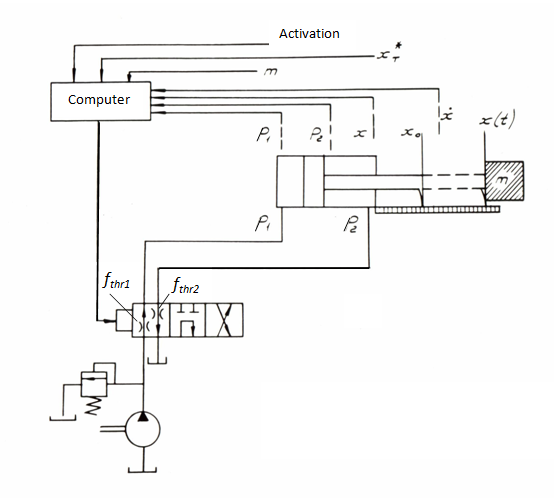
Fig. 2. For circuit diagram of a power hydraulic drive with throttle regulation (Fig. 2) the similar problem of formation of control algorithm for drive of movement is maybe stated as follows.To provide control 
for a drive of movement of a cargo mass
m
from a point
x
0
in a point
x
Т
*
for minimal
time
Т
at similar (1) and (2) restrictions and
where 
– the maximal areas of through passage windows of throttle operating arrangements.
|
